MIPLIB 2010


Tree set

[Return to complete MIPLIB 2010 problem list]

Click here for legend of abbreviations and links to subsets

Status Name Sets C Rows Cols NZs Int Bin Con Objective AGG VBD PAR PAC COV CAR EQK BIN IVK KNA IKN M01 GEN
Easy blp-ar98 T MBP 1128 16021 200601 15806 215 6205.21  X   X               X  
Easy csched007 T MBP 351 1758 6379 1457 301 351X X X                 X  
Easy csched008 RT MBP 351 1536 5687 1284 252 173X X X                 X  
Easy enlight15 T IP 225 450 1290 225 225 69                        X
Easy enlight16 IT IP 256 512 1472 256 256 Infeasible                         X
Easy glass4 BT MBP 396 322 1815 302 20 1.20001e+09  X X                 X  
Easy gmu-35-40 BT MBP 424 1205 4843 1200 5 -2.40673e+06  X   X               X  
Easy gmu-35-50 T MBP 435 1919 8643 1914 5 -2.60796e+06  X   X               X  
Easy go19 CTBP 441 441 1885 441 84        X       X        
Easy k16x240 T MBP 256 480 960 240 240 10674  X                   X  
Easy leo1 T MBP 593 6731 131218 6730 1 4.04228e+08  X   X X       X     X  
Easy markshare_5_0 T MBP 5 45 203 40 5 1                      X  
Easy maxgasflow CT MBP 7160 7437 19717 2456 4981 -4.45658e+07X X                   X  
Easy mc11 T MBP 1920 3040 6080 1520 1520 11689  X                   X  
Easy momentum1 CT MBP 42680 5174 103198 2349 2825 109143X X X           X     X  
Easy neos-1426635 T MBP 796 520 3400 260 260 -176  X   X               X  
Easy neos-1426662 T MBP 1914 832 8048 416 416 -44  X   X               X  
Easy neos-1436709 T MBP 1417 676 6214 338 338 -128  X                   X  
Easy neos-1440460 T MBP 989 468 4302 234 234 -179.25  X                   X  
Easy neos-1442119 T MBP 1524 728 6692 364 364 -181  X                   X  
Easy neos-1442657 T MBP 1310 624 5736 312 312 -154.5  X                   X  
Easy neos15 T MBP 552 792 1766 160 632 80598.4X X                   X  
Easy neos-1616732 TBP 1999 200 3998 200 159  X                      
Easy neos-1620770 TBP 9296 792 19292 792 9  X X                    
Easy neos16 T IP 1018 377 2801 41 336 446  X                 X   X
Status Name Sets C Rows Cols NZs Int Bin Con Objective AGG VBD PAR PAC COV CAR EQK BIN IVK KNA IKN M01 GEN
Easy neos-820146 ITBP 830 600 3225 600 Infeasible       X X X     X        
Easy neos-820157 ITBP 1015 1200 4875 1200 Infeasible   X X X X X     X        
Easy neos-826650 T MBP 2414 5912 20440 5792 120 29X   X X   X   X X     X  
Easy neos-826841 T MBP 2354 5516 18460 3488 2028 29.0082X   X     X   X       X  
Hard neos-847302 T MBP 609 737 9566 729 8 4  X X X               X  
Easy neos858960 ITBP 132 160 2770 160 Infeasible   X       X     X        
Easy neos-911880 T MBP 83 888 2568 840 48 54.76    X                 X  
Easy neos-942830 T MBP 803 882 13290 834 48 16X   X X         X     X  
Easy nobel-eu-DBE T MBP 879 3771 11313 1639 2132 608910X               X     X  
Easy noswot BT MIP 182 128 735 25 75 28 -41  X                 X   X
Open ns1456591 CT MIP 1997 8399 199862 19 8000 380 ?     X     X       X   X X
Easy ns1766074 BIT MIP 182 100 666 90 10 Infeasible                     X   X
Easy ns2081729 T MBP 1190 661 5680 600 61 9X X                   X  
Easy ns894788 TBP 2279 3463 14381 3463 7  X       X     X     X  
Easy p2m2p1m1p0n100 ITBP 1 100 100 100 Infeasible                   X   X  
Easy p80x400b T MBP 480 800 1600 400 400 39667X X                   X  
Easy pg T MBP 125 2700 5200 100 2600 -8674.34                      X  
Easy pigeon-10 BT MBP 931 490 8150 400 90 -9000          X     X X   X  
Easy pigeon-11 T MBP 1123 572 9889 473 99 -10000          X     X X   X  
Easy pigeon-12 CT MBP 1333 660 11796 552 108 -11000          X     X X   X  
Easy ran14x18-disj-8 T MBP 447 504 10277 252 252 3712  X     X       X     X  
Easy ran14x18 T MBP 284 504 1008 252 252 3712  X                   X  
Easy reblock166 TBP 17024 1660 39442 1660 -6.00052e+08  X               X      
Easy timtab1 BT MIP 171 397 829 107 64 226 764772                      X X
Easy umts T MIP 4465 2947 23016 72 2802 73 3.00903e+07  X X   X X     X     X X
Status Name Sets C Rows Cols NZs Int Bin Con Objective AGG VBD PAR PAC COV CAR EQK BIN IVK KNA IKN M01 GEN
Easy wachplan T IP 1553 3361 89361 1 3360 -8  X       X     X     X X
Hard wnq-n100-mw99-14 CTBP 656900 10000 1333400 10000 259  X     X       X        
Status Name Sets C Rows Cols NZs Int Bin Con Objective AGG VBD PAR PAC COV CAR EQK BIN IVK KNA IKN M01 GEN

Legend

Problem Status

Easy Easy - instance can be solved within one hour using a commercial solver
Hard Hard - instance has been solved, but is not considered easy
Open Open - optimal solution to instance is unknown

Instance Set List

BBenchmark set
CChallenge set
IInfeasible set
PPrimal set
UUnstable set
R Reoptimize set
T Tree set
XXXL - extra large instances

Problem Type List

BPBinary Program - All variables are binary
IP Integer Program - All variables are integer
MBP Mixed Binary Program - All variables are binary or continuous
MIPMixed Integer Program - Variables can be integer or continuous

Note: The problem types are used to partition the instances. Instances that match more than one type are grouped into the least general set.

Problem Feasibility List

Feasible Problems - a feasible solution is known
Infeasible Problems - the problem was proven to be infeasible
Unknown Feasiblility - no feasible solution is know, but the problem was not proven to be infeasible

Constraint Type Legend

AGGAggregation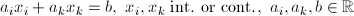
VBDVariable Bound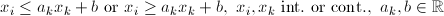
PARSet Partition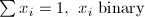
PACSet Packing
COVSet Cover
CARCardinality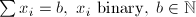
EQKEquality Knapsack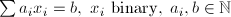
BINBin Packing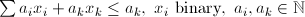
IVKInvariant Knapsack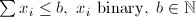
KNAKnapsack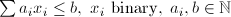
IKNInteger Knapsack
M01Mixed Binary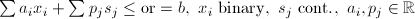
GENGeneralAll other constraint types

Note: If a constraint matches more than one type, it is counted for the one with highest priority (lowest number).
Scaling and negation of binary are applied to match constraint types.

Last Update July 31, 2019 by Gerald Gamrath
© 2019 by Konrad-Zuse-Zentrum für Informationstechnik Berlin (ZIB)
Imprint