MIPLIB 2010

IP - Pure integer programs (not contained in BP)

[Return to complete MIPLIB 2010 problem list]

Click here for legend of abbreviations and links to subsets

Status	Name	Sets	C	Rows	Cols	NZs	Int	Bin	Con	Objective	AGG	VBD	PAR	PAC	COV	CAR	EQK	BIN	IVK	KNA	IKN	M01	GEN
	30n20b8	B	IP	576	18380	109706	7344	11036		302		X	X										X
	d10200	C	IP	947	2000	57637	1267	733		12430			X						X	X
	d20200	C	IP	1502	4000	189389	819	3181		?			X						X	X
	enlight13	B	IP	169	338	962	169	169		71													X
	enlight14	B I	IP	196	392	1120	196	196		Infeasible													X
	enlight15	T	IP	225	450	1290	225	225		69													X
	enlight16	I T	IP	256	512	1472	256	256		Infeasible													X
	enlight9	I	IP	81	162	450	81	81		Infeasible													X
	lectsched-1	P	IP	50108	28718	310792	482	28236		0	X	X									X		X
	lectsched-1-obj	C	IP	50108	28718	310792	482	28236		?	X	X									X		X
	lectsched-2	P	IP	30738	17656	186520	369	17287		0	X	X									X		X
	lectsched-3	P	IP	45262	25776	279967	457	25319		0	X	X									X		X
	lectsched-4-obj	B	IP	14163	7901	82428	236	7665		4	X	X									X		X
	mzzv11	B	IP	9499	10240	134603	251	9989		-21718		X	X	X	X	X			X	X		X	X
	neos-1224597	P	IP	3276	3395	25090	245	3150		-428		X	X	X		X			X	X		X	X
	neos16	T	IP	1018	377	2801	41	336		446		X									X		X
	neos-555424	P	IP	2676	3815	15667	15	3800		1.2868e+06		X			X		X		X			X	X
	neos-686190	B	IP	3664	3660	18085	60	3600		6730		X	X		X				X				X
	ns1854840	C	IP	143616	135754	856994	474	135280		?		X				X			X				X
	ns1952667	P	IP	41	13264	335643	13264			0											X		X
	nu120-pr3	C	IP	2210	8601	25986	61	8540		28130	X	X	X			X							X
	nu60-pr9	C	IP	2220	7350	22176	42	7308		24940	X	X	X			X							X
	pw-myciel4	B	IP	8164	1059	17779	1	1058		10		X	X										X
	rococoB10-011000	C	IP	1667	4456	16517	136	4320		19449		X	X			X							X
	rococoC10-001000	B	IP	1293	3117	11751	124	2993		11460		X	X			X							X
Status	Name	Sets	C	Rows	Cols	NZs	Int	Bin	Con	Objective	AGG	VBD	PAR	PAC	COV	CAR	EQK	BIN	IVK	KNA	IKN	M01	GEN
	rococoC11-011100	C R	IP	2367	6491	30472	166	6325		20889	X	X	X			X			X				X
	rococoC12-111000	C	IP	10776	8619	48920	187	8432		?		X	X			X							X
	sp98ir	B	IP	1531	1680	71704	809	871		2.19677e+08		X						X		X		X	X
	tw-myciel4	C	IP	8146	760	27961	1	759		10	X	X			X				X				X
	wachplan	T	IP	1553	3361	89361	1	3360		-8		X				X			X			X	X
Status	Name	Sets	C	Rows	Cols	NZs	Int	Bin	Con	Objective	AGG	VBD	PAR	PAC	COV	CAR	EQK	BIN	IVK	KNA	IKN	M01	GEN

Legend

Problem Status

Easy Easy - instance can be solved within one hour using a commercial solver
Hard Hard - instance has been solved, but is not considered easy
Open Open - optimal solution to instance is unknown

Instance Set List

B	Benchmark set
C	Challenge set
I	Infeasible set
P	Primal set
U	Unstable set
R	Reoptimize set
T	Tree set
X	XXL - extra large instances

Problem Type List

BP	Binary Program - All variables are binary
IP	Integer Program - All variables are integer
MBP	Mixed Binary Program - All variables are binary or continuous
MIP	Mixed Integer Program - Variables can be integer or continuous

Note: The problem types are used to partition the instances. Instances that match more than one type are grouped into the least general set.

Problem Feasibility List

Feasible Problems - a feasible solution is known
Infeasible Problems - the problem was proven to be infeasible
Unknown Feasiblility - no feasible solution is know, but the problem was not proven to be infeasible

Constraint Type Legend

AGG	Aggregation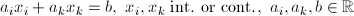
VBD	Variable Bound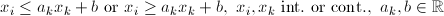
PAR	Set Partition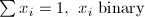
PAC	Set Packing
COV	Set Cover
CAR	Cardinality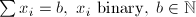
EQK	Equality Knapsack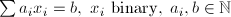
BIN	Bin Packing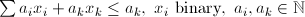
IVK	Invariant Knapsack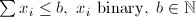
KNA	Knapsack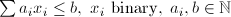
IKN	Integer Knapsack
M01	Mixed Binary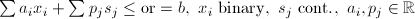
GEN	General	All other constraint types

Note: If a constraint matches more than one type, it is counted for the one with highest priority (lowest number).
Scaling and negation of binary are applied to match constraint types.