MIPLIB 2010

XXL - extra large set

[Return to complete MIPLIB 2010 problem list]

Click here for legend of abbreviations and links to subsets

Status	Name	Sets	C	Rows	Cols	NZs	Int	Bin	Con	Objective	AGG	VBD	PAR	PAC	COV	CAR	EQK	BIN	IVK	KNA	IKN	M01	GEN
	hawaiiv10-130	C U X	MBP	1388052	685130	183263061		578444	106686	?	X	X	X	X		X			X			X
	in	C R X	MBP	1526202	1449074	6811639		1489	1447585	58	X	X										X
	ivu06-big	C R X	BP	1177	2277736	23125770		2277736		?			X
	mspp16	B X	BP	561657	29280	27678735		29280		363		X	X	X	X				X	X		X
	ns1663818	C X	BP	172017	124626	20433649		124626		86		X	X	X					X	X		X
	pb-simp-nonunif	C X	BP	1451912	23848	4366648		23848		?	X	X			X				X
	rmine21	C X	BP	1441651	162547	3514884		162547		?		X										X
	rmine25	C X	BP	2953849	326599	7182744		326599		?		X										X
	splan1	C U X	MIP	572800	1317382	5233840	1978	90810	1224594	?	X	X		X		X			X	X	X	X	X
	zib01	C X	BP	5887041	12471400	49877768		12471400		?			X	X		X
	zib02	C I X	BP	9049868	37709944	146280582		37709944		Infeasible		X	X	X		X
Status	Name	Sets	C	Rows	Cols	NZs	Int	Bin	Con	Objective	AGG	VBD	PAR	PAC	COV	CAR	EQK	BIN	IVK	KNA	IKN	M01	GEN

Legend

Problem Status

Easy Easy - instance can be solved within one hour using a commercial solver
Hard Hard - instance has been solved, but is not considered easy
Open Open - optimal solution to instance is unknown

Instance Set List

B	Benchmark set
C	Challenge set
I	Infeasible set
P	Primal set
U	Unstable set
R	Reoptimize set
T	Tree set
X	XXL - extra large instances

Problem Type List

BP	Binary Program - All variables are binary
IP	Integer Program - All variables are integer
MBP	Mixed Binary Program - All variables are binary or continuous
MIP	Mixed Integer Program - Variables can be integer or continuous

Note: The problem types are used to partition the instances. Instances that match more than one type are grouped into the least general set.

Problem Feasibility List

Feasible Problems - a feasible solution is known
Infeasible Problems - the problem was proven to be infeasible
Unknown Feasiblility - no feasible solution is know, but the problem was not proven to be infeasible

Constraint Type Legend

AGG	Aggregation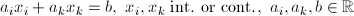
VBD	Variable Bound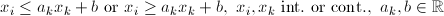
PAR	Set Partition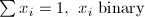
PAC	Set Packing
COV	Set Cover
CAR	Cardinality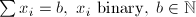
EQK	Equality Knapsack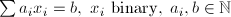
BIN	Bin Packing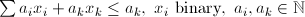
IVK	Invariant Knapsack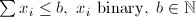
KNA	Knapsack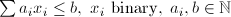
IKN	Integer Knapsack
M01	Mixed Binary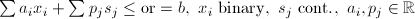
GEN	General	All other constraint types

Note: If a constraint matches more than one type, it is counted for the one with highest priority (lowest number).
Scaling and negation of binary are applied to match constraint types.